The Curta: A Mechanical Calculating Machine
A mechanical calculator based on the Leibniz Wheel
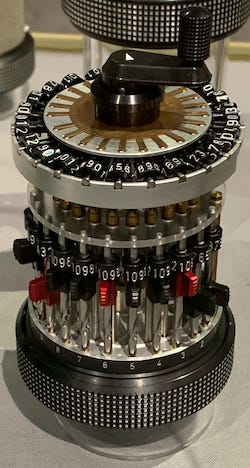
You are asked to multiply together 731 and 379. How do you do it? Maybe you ask Alexa, Siri, or Google. You might reach for your phone. Maybe you have an abandoned calculator laying around. Or, perhaps you momentarily think about pulling out paper and pencil, before laughing at the ridiculousness of the thought. But, what you probably don't reach for is a pocket-sized canister containing a mechanical calculating machine. But not all that long ago, in the 1950s, you might have.
Produced between 1948 and 1972 by Contina AG, the Curta was such a pocket-sized mechanical calculating machine. Invented by Curt Herzstark in 1936 and improved on over subsequent years, it was first produced after World War II in 1948, in the small principality of Lichtenstein.
Mechanical calculating machines, or concepts relating to them go back centuries. In essence, these devices were the precursors to our modern day computers. One of the most well known is the Stepped Reckoner from Gottfried Leibniz in the 17th century. This device was the first to be able to perform all four arithmetic operations (add, subtract, multiply, and divide). It had an 8 digit input and an accumulator that could hold 16 decimal digits. An accumulator! That's like a shitty 17th century version of RAM! However, the key innovation was the Leibniz wheel — a cylinder with stepped teeth, or ledges which were combined with a counting wheel (think music box, sort of). The Leibniz wheel was used in mechanical calculating machines until the electric calculator became available in the mid-1970s. And, it was used by Curt Herzstark in the Curta.
So, how would one multiply 731 and 379 on a Curta calculating machine? The Curta displays the result in a counter (an accumulator) around the top of the device. First the the number which is to be multiplied (731) is set into the counter using setting pins on the side of the device. One can then multiply the set number by turning the crank 379 times. Conveniently, the Curta allows the user to move the crank carriage in a way which shifts decimal places so that multiplying by 379 is done by turning the crank 9 times, moving the carriage one place (10), turning the crank 7 times, moving the crank one more place (100) and turning the crank 3 times. Somewhat laborious. But, faster and more accurate than paper and pencil!
What's fun about the Curta is how it represents the end of the line for centuries of mathematicians dreaming about building logical or thinking machines, as these were superseded by the transistor and our modern computers. But within these mechanical calculating machines there are a lot of ideas that are still with us in modern computing today. For example, in 1679, Leibniz describes how a "[binary] calculus could be implemented by a machine (without wheels) in the following manner, easily to be sure and without effort. A container shall be provided with holes in such a way that they can be opened and closed. They are to be open at those places that correspond to a 1 and remain closed at those that correspond to a 0. Through the opened gates small cubes or marbles are to fall into tracks, through the others nothing. It [the gate array] is to be shifted from column to column as required.” 🤯. Four centuries ago, Leibniz had already figured out that one could perform all arithmetic operations using only binary numbers 0 and 1. In essence, he's described some of the basic components of modern-day computers. Though, we've replaced the marbles and gravity with voltage gradients and electrons.
It's a humbling reminder that we're rarely as original as we think we are. Our greatest scientists, mathematicians, or engineering heroes all build on top of thinking and work done by their predecessors—and some of that thinking goes back far further than you'd think.
The Liechtenstein National Museum (Landesmuseum) in Vaduz currently has a special exhibition until August 28th, 2021 on the Curta calculator. Die Curta – Made in Liechtenstein describes Curt Herzstark's journey to bring the Curta to market, as well as the history of the company, Contina AG, which produced it. There's also this fun online simulator which let's you play with a Curta, as well as a video on how it was operated.
Sources
Die Curta – Made in Liechtenstein, Special exhibition at the Lichtenstein Landesmuseum, visited 13.06.2021.
https://www.ias.edu/ideas/2012/george-dyson-ecphttps://en.wikipedia.org/wiki/Leibniz_wheel
https://en.wikipedia.org/wiki/Stepped_reckoner
https://en.wikipedia.org/wiki/Curta
https://history-computer.com/curta-calculator-history-of-the-curta-mechanical-computer-calculator/
https://history-computer.com/gottfried-leibniz/
This article is part of my 30 days / 30 articles challenge where I've attempted to write thirty articles within thirty days.